JEE Main 2020 Maths paper for September 4 Shift 1 with solutions are available on this page. Students can view or download the question paper and analyze the different aspects of the JEE Main exam paper including exam pattern, weightage of marks, difficulty level and more. Students can also practice solving the question and the solutions which have been prepared by our experts will help them understand the answers more clearly. These solutions are available in PDF format and can be accessed for free.

September 4 Shift 1 - Maths

Question 1.Let y = y(x) be the solution of the differential equation, xy'-y = x2(xcosx+sinx),x > 0. If y(π) = π, then y’’(π/2)+y(π/2) is equal to:

  1. a) 2+(π/2)+(π2/4)
  2. b) 2+(π/2)
  3. c) 1+(π/2)
  4. d) 1+(π/2)+ (π2/4)

Solution:

  1. xy’-y = x2(x cosx + sinx) x > 0, y(π) =π

    y'-(1/x)y = x(x cosx+sin x)

    I.F =

    \(e^{-\int\frac{1}{x}dx }\)

    = e- ln x

    = 1/x

    y(1/x) = ∫(1/x)x(x cos x+sin x) dx

    (y/x) = ∫(x cos x+ sin x) dx

    (y/x) = ∫ (d/dx)(x sin x) dx

    (y/x) = x sin x+C

    y = x2 sin x+Cx

    x = π, y = π

    π = πC

    C = 1

    y = x2 sin x+x

    y(π/2) = (π2/4)+(π/2)

    y' = 2x sinx+x2cosx+1

    y’’ = 2sinx+2xcosx+2x cosx-x2sinx

    y’’(π/2) = 2-(π2/4)

    y(π/2)+ y’’(π/2) = 2+π/2

    Answer:(b)

Question 2. The value of

\(\sum_{r=0}^{20} \: ^{50-r}C_{6}\)
is equal to:

  1. a) 51C7-30C7
  2. b) 51C7+30C7
  3. c) 50C7-30C7
  4. d) 50C6-30C6

Solution:

  1. \(\sum_{r=0}^{20} \: ^{50-r}C_{6}\)

    = 50C6+49C6+48C6+…+31C6+30C6

    Add and subtract 30C7

    Using nCr+ nCr-1 = n+1Cr

    30C6+30C7 = 31C7

    31C6+31C7 = 32C7

    Similarly solving

    51C7-30C7

    Answer: (a)

Question 3. Let [t] denote the greatest integer ≤ t. Then the equation in x, [x]2+2[x+2]-7 = 0 has:

  1. a) exactly four integral solutions.
  2. b) infinitely many solutions.
  3. c) no integral solution.
  4. d) exactly two solutions.

Solution:

  1. [x]2+2[x+2]-7 = 0

    [x]2 +2[x]-3 = 0

    let [x] = y

    y2+3y-y -3 = 0

    (y -1)(y+3) = 0

    [x] = 1 or [x] = -3

    x ∈ [1,2) or x ∈ [-3,-2)

    Answer: (b)

Question 4. Let P(3, 3) be a point on the hyperbola, (x2/a2)-(y2/b2) = 1. If the normal to it at P intersects the x-axis at (9, 0) and e is its eccentricity, then the ordered pair (a2, e2) is equal to:

  1. a) (9, 3)
  2. b) (9/2, 2)
  3. c) (9/2, 3)
  4. d) (3/2, 2)

Solution:

  1. (x2/a2)-(y2/b2) = 1

    Point P(3, 3) on hyperbola.

    (9/a2)-(9/b2) = 1 ..(i)

    Equation of normal (a2x/3)+(b2y)/3 = a2e2

    At x axis y = 0

    a2x/3 = a2e2

    x = 3e2 = 9

    3e2 = 9

    e2 = 3

    e = √3

    e2 = 1+b2/a2 = 3

    b2 = 2a2 ..(ii)

    Put in equation 1

    (9/a2)-(9/2a2) = 1

    (9/2a2) = 1

    a2 = 9/2

    (a2, e2) = (9/2,3)

    Answer: (c)

Question 5. Let (x2/a2)+(y2/b2) = 1 (a>b) be a given ellipse, length of whose latus rectum is 10. If its eccentricity is the maximum value of the function, Φ(t) = (5/12)+t-t2, then a2+b2 is equal to:

  1. a) 135
  2. b) 116
  3. c) 126
  4. d) 145

Solution:

  1. L.R = 2b2/a = 10 ..(i)

    Φ(t) = (5/12)-(t-1/2)2+(1/4)

    = (8/12)-(t-1/2)2

    Φ(t)max = 2/3 = e

    e2 = 1-(b2/a2)

    = 4/9

    b2/a2 = 5/9

    From (i)

    b2/a.a = 5/9

    5/a = 5/9

    a = 9

    b2 = 45

    a2+b2 = 81+45 = 126

    Answer: (c)

Question 6. Let

\(f(x) = \int \frac{\sqrt{x}}{(1+x)^{2}}dx\)
, x≥0. Then f(3)-f(1) is equal to:

  1. a) (-π/6)+(1/2)+(√3/4)
  2. b) (π/6)+(1/2)-(√3/4)
  3. c) (-π/12)+(1/2)+(√3/4)
  4. d) (π/12)+(1/2)-(√3/4)

Solution:

  1. \(f(x) = \int \frac{\sqrt{x}}{(1+x)^{2}}dx\)

    Substituting x = tan2t

    dx = 2tan t sec2t dt

    f(x) =

    \(\int \frac{\tan t. 2\tan t\sec ^{2}t}{\sec ^{4}t}dt\)

    f(x) = 2∫sin2t dt

    x = 3 t = π/3

    x = 1 t = π/4

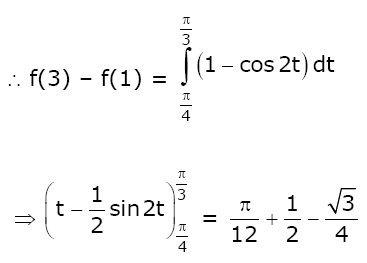
    JEE Main 2020 Paper With Solution Maths Sept 4 Shift 1

    Answer: (d)

Question 7. If 1+(1-22×1)+(1-42×3)+(1-62×5)+......+(1-202×19) = α - 220β, then an ordered pair (α, β) is equal to:

  1. a) (10,97)
  2. b) (11,103)
  3. c) (11,97)
  4. d) (10,103)

Solution:

  1. Tn = 1-(2n)2(2n-1)

    = 1-4n2(2n-1)

    = 1-8n3+4n2

    Sn =

    \(\sum_{n=1}^{10}T_{n}\)

    = n-∑8n3+∑4n2

    = n-8×(n2(n+1)2/4)+4n(n+1)(2n+1)/6

    S10 = 10-2×100×121 +(2/3)×10×11×21

    = 10-24200+1540

    = 10-22660

    Sum of series = 11-220×103

    = α - 220β

    α = 11

    β = 103

    Answer: (b)

Question 8. The integral

\(\int \left ( \frac{x}{x\sin x+\cos x} \right )^{2}dx\)
is equal to: (where C is a constant of integration)

  1. a) tan x-(x sec x/(x sin x+cos x))+C
  2. b) sec x-(x tan x/(x sin x+cos x))+C
  3. c) sec x+(x tan x/(x sin x+cos x))+C
  4. d) tan x+(x sec x/(x sin x+cos x))+C

Solution:

  1. \(\int \left ( \frac{x}{x\sin x+\cos x} \right )^{2}dx\)

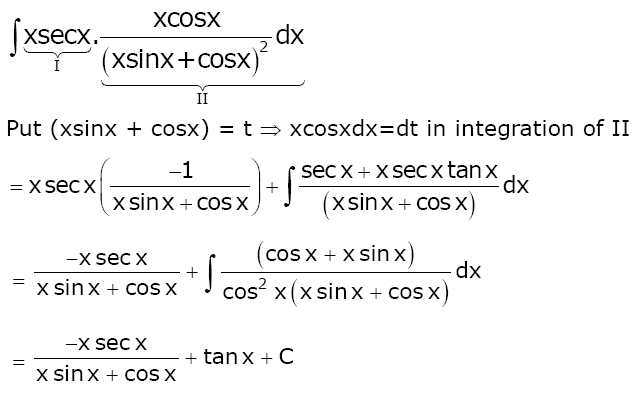
    JEE Main 2020 Papers With Solutions Sept 4 Maths Shift 1

    = tan x-(x sec x/(x sin x+cos x))+C

    Answer: (a)

Question 9. Let f(x)= |x-2| and g(x) = f(f(x)) ,x∈0,4]. Then

\(\int_{0}^{3}(g(x)-f(x))dx\)
is equal to:

  1. a) 1/2
  2. b) 0
  3. c) 1
  4. d) 3/2

Solution:

  1. f(x) = |x-2| =

    \(\left\{\begin{matrix} x-2, & x\geq 2\\ 2-x, & x< 2 \end{matrix}\right.\)

    g(x) = ||x-2|-2| =

    \(\left\{\begin{matrix} \left | x-4 \right |, & x\geq 2\\ \left | x \right |, & x< 2 \end{matrix}\right.\)

    =

    \(\left\{\begin{matrix} 4-x, & x\in [2,4)\\ x-4 ,&x\geq 4 \\ x, & x\in [0,2) \end{matrix}\right.\)

    \(\int_{0}^{3}(g(x)-f(x))dx\)

    =

    \(\int_{0}^{3}g(x)dx-\int_{0}^{3}f(x)dx\)

    = [(1/2)×2×2+1+(1/2)×1×1]-[(1/2)×2×2+(1/2)×1×1)]

    = (7/2)-(5/2)

    = 1

    JEE Main 2020 Maths Papers With Solutions Sept 4 Shift 1

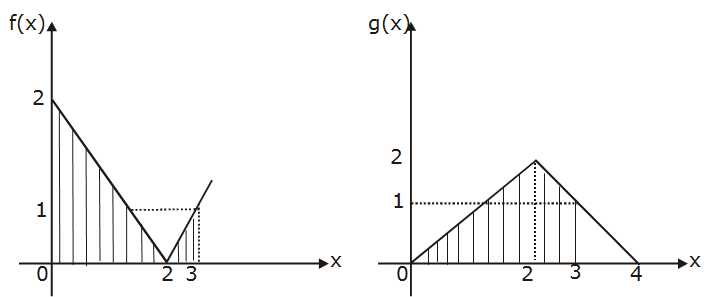
    Answer: (c)

Question 10. Let x0 be the point of local maxima of f(x) =

\(\vec{a}.(\vec{b}\times \vec{c})\)
where
\(\vec{a}= x\hat{i}-2\hat{j}+3\hat{k}\)
,
\(\vec{b}= -2\hat{i}+x\hat{j}-\hat{k}\)
and
\(\vec{c}= 7\hat{i}-2\hat{j}+x\hat{k}\)
. Then the value of
\(\vec{a}.\vec{b}+\vec{b}.\vec{c}+\vec{c}.\vec{a}\)
at x = x0 is:

  1. a) -22
  2. b) -4
  3. c) -30
  4. d) 14

Solution:

  1. \(\vec{a}.(\vec{b}\times \vec{c})\)

    =

    \(\begin{vmatrix} x & -2 & 3\\ -2 & x & -1\\ 7 & -2 & x \end{vmatrix}\)

    = x(x2-2)+2(-2x+7)+ 3(4-7x)

    = x3-2x- 4x+14+12-21x

    f(x) = x3- 27x+26

    f’(x) = 3x2-27 = 0

    x = 3

    f’’(x) = 6x

    at x = 3, f” (3) = 6×3= 18 > 0

    at x = -3, f” (-3) = 6×3 = -18 < 0

    Max at x0 = -3

    \(\vec{a}= (-3,-2,3)\)
    ,
    \(\vec{b}= (-2,-3,-1)\)
    ,
    \(\vec{c}= (7,-2,-3)\)

    So

    \(\vec{a}.\vec{b}+\vec{b}.\vec{c}+\vec{c}.\vec{a}\)

    = 6+6-3-14+6+3-21+4-9

    = 25-47

    = -22

    Answer: (a)

Question 11.A triangle ABC lying in the first quadrant has two vertices as A(1,2) and B(3,1). If BAC = 900 and ar(ΔABC) = 5√5 s units, then the abscissa of the vertex C is:

  1. a) 1+√5
  2. b) 1+2√ 5
  3. c) 2√5-1
  4. d) 2+√5

Solution:

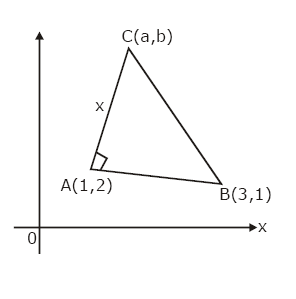
  1. JEE Main 2020 Maths Papers Sept 4 Shift 1 With Solutions

    AB = √(4+1) = √5

    (1/2)×√5×x = 5√5

    x = 10

    mAB = -1/2

    mAC = 2 = tanθ

    Since sinθ = 2/√5

    cosθ = 1/√5

    by parametric co-ordinates

    a = 1+ xcosθ = 1+10×1/√5

    = 1+2√5

    Answer: (b)

Question 12.Let f be a twice differentiable function on (1,6). If f(2) = 8, f’(2) = 5, f’(x) ≥1 and f’’(x) ≥4, for all x ∈ (1,6), then:

  1. a) f(5)+f’(5) ≥28
  2. b) f’(5)+f’’(5) ≤20
  3. c) f(5) ≤10
  4. d) f(5)+f’(5) ≤26

Solution:

  1. f(2) = 8, f’(2) = 5, f’(x) ≥1 and f’’(x) ≥4,

    x∈(1,6)

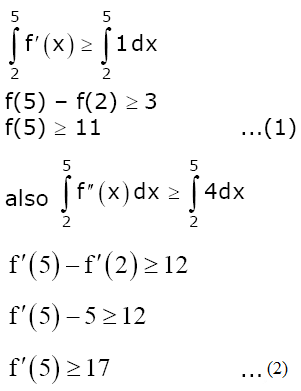
    JEE Main Solution 2020 Maths Papers Sept 4 Shift 1

    adding (1) and (2) we get,

    f(5)+f’(5) ≥28

    Answer: (a)

Question 13.Let α and β be the roots of x2-3x+p = 0 and 1/γ and 1/δ be the roots of x2-6x+q = 0. If α, β, γ, δ form a geometric progression. Then ratio (2q+p): (2q-p) is:

  1. a) 33 :31
  2. b) 9 : 7
  3. c) 3 : 1
  4. d) 5 : 3

Solution:

  1. Roots of x2-3x+p = 0 are α and β.

    The roots of x2-6x+q = 0 are γ and δ.

    α + β = 3

    γ + δ = 6

    α = a , β = ar, γ = ar2, δ = ar3

    a(1+r) = 3 ...(i)

    ar2(1+r) = 6 ...(ii)

    Divide (ii) by (i)

    r2 = 2

    α.β = p = a2r

    γ.δ = q = a2r5

    (2q+p)/(2q-p) = (2r4+1)/( 2r4-1)

    = (2×22+1)/( 2×22-1) = 9/7

    Answer: (b)

Question 14. Let u = (2z+i)/(z-ki), z = x+iy and k>0. If the curve represented by Re(u) +Im(u) =1 intersects the y-axis at the points P and Q where PQ =5, then the value of k is:

  1. a) 4
  2. b) 1/2
  3. c) 2
  4. d) 3/2

Solution:

  1. u = (2z+i)/(z-ki)

    z = x+iy

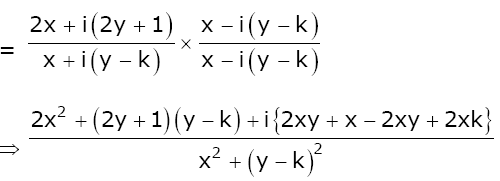
    JEE Main Solution Sept 4 Shift 1 2020 Maths Papers

    Re(u)+Img(u) = 1

    2x2+(2y+1)(y -k )+x+2xk = x2 + (y-k)2

    at y - axis, x = 0

    (2y +1)(y- k) = (y- k )2

    2y2 +y- 2yk- k = y2+k2-2yk

    Roots of y2+y-(k +k2) = 0 are y1 and y2

    Diff. of roots = 5

    √(1+4k+4k2) = 5

    4k2+4k = 24

    k2+k-6 = 0

    (k +3)(k-2) = 0

    k = 2

    Answer: (c)

Question 15. If A =

\(\begin{bmatrix} \cos \theta & i\sin \theta \\ i\sin \theta & \cos \theta \end{bmatrix}\)
, (θ = π/24) and A5 =
\(\begin{bmatrix} a & b\\ c& d \end{bmatrix}\)
, where i = √-1, then which one of the following is not true?

  1. a) a2-d2 = 0
  2. b) a2-c2 = 1
  3. c) 0 ≤a2+b2 ≤1
  4. d) a2-b2 = 1/2

Solution:

  1. A =

    \(\begin{bmatrix} \cos \theta & i\sin \theta \\ i\sin \theta & \cos \theta \end{bmatrix}\)

    A2 =

    \(\begin{bmatrix} \cos \theta & i\sin \theta \\ i\sin \theta & \cos \theta \end{bmatrix} \begin{bmatrix} \cos \theta & i\sin \theta \\ i\sin \theta & \cos \theta \end{bmatrix}\)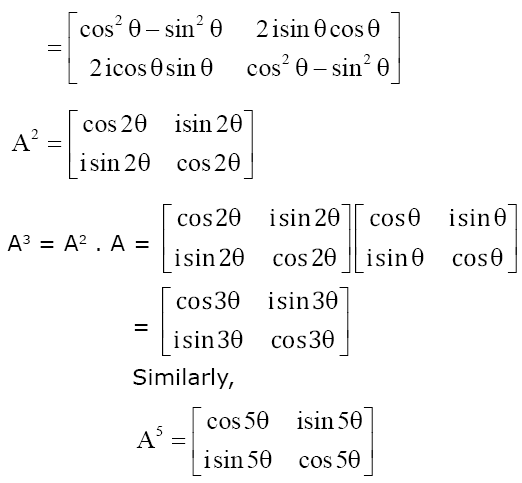

    JEE Main Sept 4 Shift 1 2020 Solved Maths Papers

    a = d = cos (5θ)

    b = c = i sin (5θ)

    a2-b2 = cos25θ + sin25θ

    = 1

    Answer: (d)

Question 16. The mean and variance of 8 observations are 10 and 13.5, respectively. If 6 of these observations are 5, 7, 10, 12, 14, 15, then the absolute difference of the remaining two observations is:

  1. a) 3
  2. b) 9
  3. c) 7
  4. d) 5

Solution:

  1. (5+7+10+12+14+15+x+y)/8 = 10

    x+y = 17 ..(i)

    Variance = (52+72+102+122+142+152+x2+y2)/8 -100 = 13.5

    (739+x2+y2)/8 - 100 = 13.5

    x2+y2 = 169 ..(ii)

    ∴ x = 12, y = 5

    |x-y| = 7

    Answer: (c)

Question 17. A survey shows that 63% of the people in a city read newspaper A whereas 76% read newspaper B. If x% of the people read both the newspapers, then a possible value of x can be:

  1. a) 37
  2. b) 29
  3. c) 65
  4. d) 55

Solution:

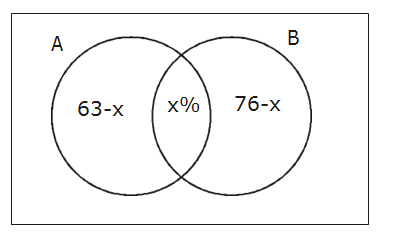
  1. Shift 1 JEE Main Sept 4 2020 Solved Maths Papers

    n(B) ≤n(A∪B) ≤n(U)

    76 ≤76+63-x ≤100

    -63 ≤-x ≤-39

    63 ≥x ≥ 39

    Answer (d)

Question 18.Given the following two statements

(S1): (q˅p)→(p ↔ ~q) is a tautology.

(S2): ~q ˄ (~p ↔ q) is a fallacy. Then:

  1. a) only (S1) is correct.
  2. b) both (S1) and (S2) are correct.
  3. c) only (S2) is correct
  4. d) both (S1) and (S2) are not correct.

Solution:

  1. Shift 1 2020 JEE Main Sept 4 Solved Maths Papers

    Answer (d)

Question 19. Two vertical poles AB = 15 m and CD = 10 m are standing apart on a horizontal ground with points A and C on the ground. If P is the point of intersection of BC and AD, then the height of P (in m) above the line AC is:

  1. a) 5
  2. b) 20/3
  3. c) 10/3
  4. d) 6

Solution:

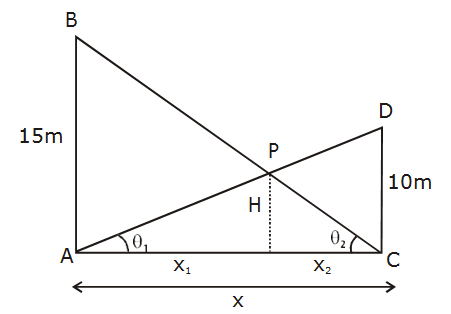
  1. Shift 1 Sept 4 JEE Main 2020 Solved Maths Papers

    Using similar triangle concept

    tan θ1 = 10/x = H/x1

    x1 = Hx/10

    tan θ2 = 15/x = H/x2

    x2 = Hx/15

    Since x1+x2 = x

    (Hx/10)+( Hx/15) = x

    15H+10H = 150

    H = 150/25 = 6 m

    Answer: (d)

Question 20. If (a+√2b cos x)( a-√2b cos y) = a2-b2, where a>b>0, then dy/dx at (π/4, π/4) is:

  1. a) (a+b)/(a-b)
  2. b) (a-2b)/(a+2b)
  3. c) (a-b)/(a+b)
  4. d) (2a+b)/(2a-b)

Solution:

  1. (a+√2b cos x)( a-√2b cos y) = a2-b2

    Differentiating both sides w.r.t.y

    -√2b sin x (dx/dy)(a-√2b cos y)+ (a+√2b cos x)(√2b sin y) = 0

    x = y = π/4

    -b(dx/dy) (a-b)+(a+b)b = 0

    dx/dy = (a+b)/(a-b)

    Answer: (a)

Question 21. Suppose a differentiable function f(x) satisfies the identity f(x+y) = f(x)+f(y)+xy2+x2y,for all real x and y. If

\(\lim_{x\to0}\frac{f(x)}{x}=1\)
then f’(3) is equal to:

    Solution:

    1. f(x+y) = f(x)+f(y)+xy2+x2y

      x = y = 0

      f(0) = 2f(0)

      f(0) = 0

      Now,

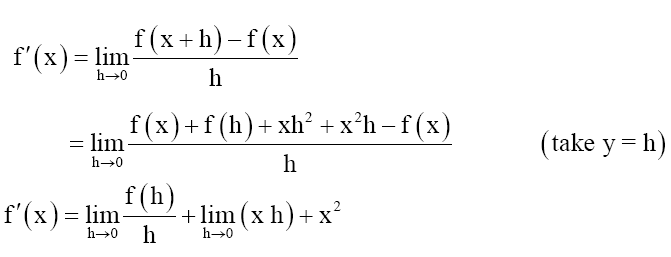
      Maths Shift 1 JEE Main Sept 4 2020 Solved Papers

      f’(x) = 1+0+x2

      f’(x) = 1+x2

      f’(3) = 10

      Answer: (10)

    Question 22.If the equation of a plane P, passing through the intersection of the planes, x+4y-z+7 = 0 and 3x+y+5z = 8 is ax+by+6z = 15 for some a, b∈R, then the distance of the point (3,2,-1) from the plane P is ….. units.

      Solution:

      1. p1+λp2 = 0

        (x +4y-z+7)+λ(3x+y+5z-8) = ax+by+6z-15

        (1+3λ)/a = (4+λ)/b = (-1+5λ)/6 = (7-8λ)/-15

        ∴ 15-75λ = 42-48λ

        -27 = 27λ

        = -1

        ∴ plane is -2x+3y-6z+15 = 0

        d = |-6+6+6+15)/√(4+9+36)|

        = 3 units

        Answer: (3)

      Question 23. If the system of equations

      x-2y+3z = 9

      2x+y+z = b

      x-7y+az = 24, has infinitely many solutions, then a-b is equal to:

        Solution:

        1. D = 0

          \(\begin{vmatrix} 1 & -2& 3\\ 2 & 1 & 1\\ 1& -7 & a \end{vmatrix}\)
          = 0

          1(a +7)+2(2a -1)+3(-14-1) = 0

          a+7+4a-2-45 = 0

          5a = 40

          a = 8

          D1 =

          \(\begin{vmatrix} 9 & -2& 3\\ b & 1 & 1\\ 24& -7 & 8 \end{vmatrix} = 0\)

          9(8+7)+2(8b-24)+3(-7b-24) = 0

          135+16b-48-21b-72 = 0

          15 = 5b

          b = 3

          Hence a - b = 8-3 = 5

          Answer: (5)

        Question 24.Let (2x2+3x+4)10 =

        \(\sum_{r =0}^{20}a_{r}x^{r}\)
        . Then a7/a13 is equal to:

          Solution:

          1. Given (2x2+3x+4)10 =

            \(\sum_{r =0}^{20}a_{r}x^{r}\)

            Replace x by 2/x in above identity:

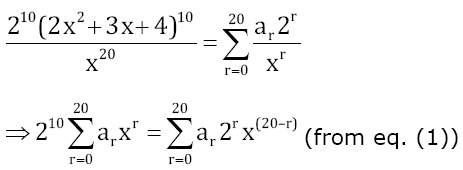
            Maths Shift 1 Sept 4 2020 JEE Main Solved Papers

            Now, comparing coefficient of x7 from both sides

            (take r = 7 in L.H.S. and r = 13 in R.H.S)

            210a7 = a13213

            a7/a13 = 23 = 8

            Answer: (8)

          Question 25.The probability of a man hitting a target is 1/10. The least number of shots required, so that the probability of his hitting the target at least once is greater than 1/4 is:

            Solution:

            1. Probability of hitting, P(H) = 1/10

              probability of missing, P(M) = 9/10

              We have, 1-(probability of all shots result in failure ) >1/4

              = 1-P(M)n > 1/4

              = 1-(9/10)n > 1/4

              (9/10)n <3/4

              n≥3

              Answer (3)

            Warning: Undefined variable $snippet_dislikes in /var/app/current/wp-content/themes/html5blank-stable/functions.php on line 2428 Warning: Undefined variable $snippet_dislikes in /var/app/current/wp-content/themes/html5blank-stable/functions.php on line 2428 Warning: Undefined variable $snippet_dislikes in /var/app/current/wp-content/themes/html5blank-stable/functions.php on line 2428 Warning: Undefined variable $snippet_dislikes in /var/app/current/wp-content/themes/html5blank-stable/functions.php on line 2428

            Video Lessons - Maths









            JEE Main 2020 Maths Paper With Solutions Sep 4 Shift 1

            JEE Main 2020 Maths Paper With Solutions Sep 4 Shift 1
            JEE Main 2020 Maths Paper With Solutions Sep 4 Shift 1
            JEE Main 2020 Maths Paper With Solutions Sep 4 Shift 1
            JEE Main 2020 Maths Paper With Solutions Sep 4 Shift 1
            JEE Main 2020 Maths Paper With Solutions Sep 4 Shift 1
            JEE Main 2020 Maths Paper With Solutions Sep 4 Shift 1
            JEE Main 2020 Maths Paper With Solutions Sep 4 Shift 1
            JEE Main 2020 Maths Paper With Solutions Sep 4 Shift 1
            JEE Main 2020 Maths Paper With Solutions Sep 4 Shift 1
            JEE Main 2020 Maths Paper With Solutions Sep 4 Shift 1
            JEE Main 2020 Maths Paper With Solutions Sep 4 Shift 1
            JEE Main 2020 Maths Paper With Solutions Sep 4 Shift 1
            JEE Main 2020 Maths Paper With Solutions Sep 4 Shift 1