CBSE Class 10 Maths Circles Notes:-Download PDF Here
A brief introduction to circles for class 10 is provided here. Get the complete description provided here to learn about the concept of the circle. Also, learn how to draw a tangent to the circle with various theorems and examples.
Introduction to Circles
For More Information On Circles, Watch The Below Videos.


To know more about Circles, visit here.
Circle and line in a plane
For a circle and a line on a plane, there can be three possibilities.
i) they can be non-intersecting
ii) they can have a single common point: in this case, the line touches the circle.
ii) they can have two common points: in this case, the line cuts the circle.
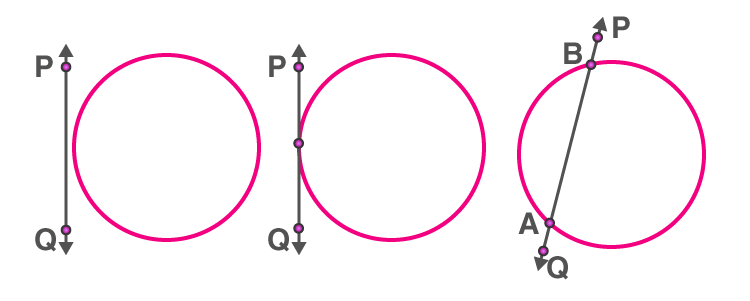
(i) Non intersecting (ii) Touching (iii) Intersecting
Tangent
A tangent to a circle is a line that touches the circle at exactly one point. For every point on the circle, there is a unique tangent passing through it.
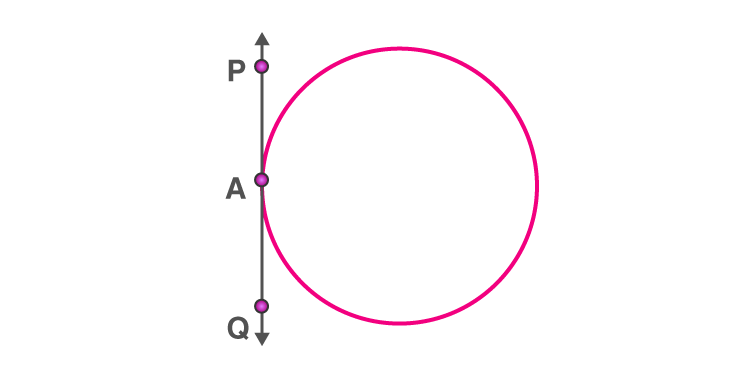
Tangent
To know more about Tangent, visit here.
Secant
A secant to a circle is a line that has two points in common with the circle. It cuts the circle at two points, forming a chord of the circle.
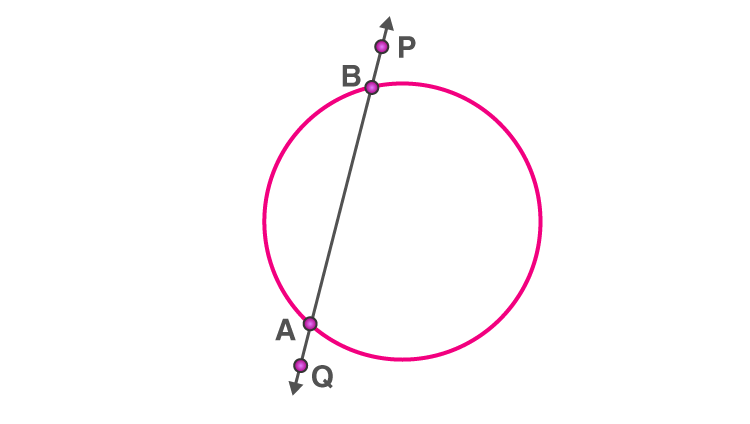
Secant
To know more about Secant, visit here.
Tangent as a special case of Secant
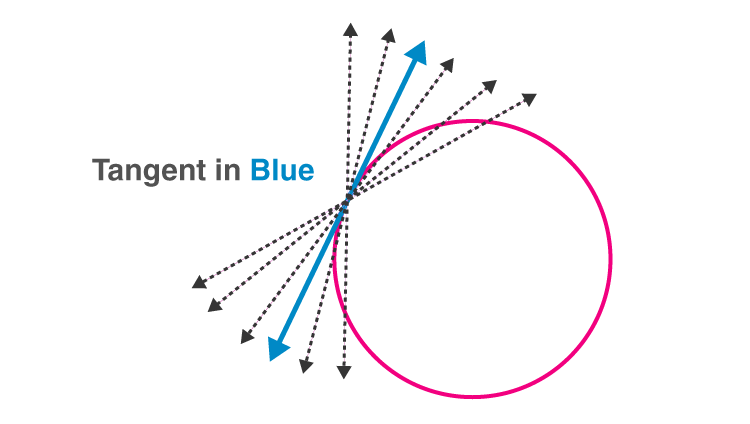
Tangent as a special case of Secant
The tangent to a circle can be seen as a special case of the secant when the two endpoints of its corresponding chord coincide.
Two parallel tangents at most for a given secant
For every given secant of a circle, there are exactly two tangents which are parallel to it and touches the circle at two diametrically opposite points.
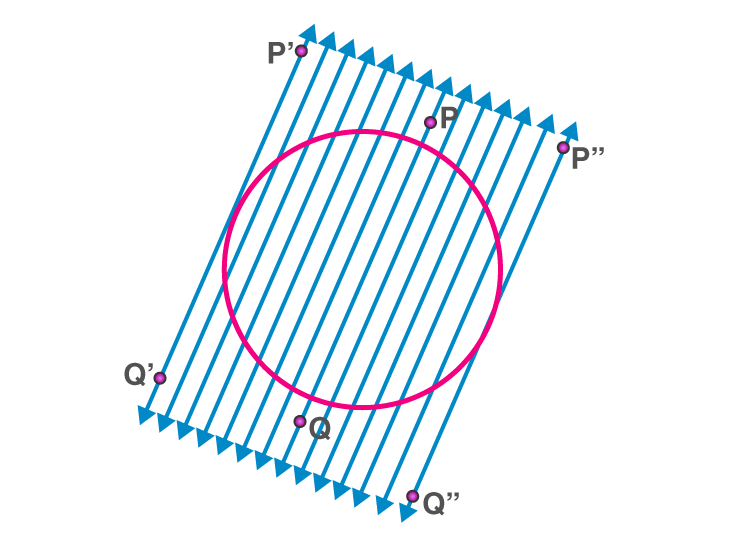
Parallel tangents
Theorems
Tangent perpendicular to the radius at the point of contact
Theorem: The theorem states that “the tangent to the circle at any point is the perpendicular to the radius of the circle that passes through the point of contact”.
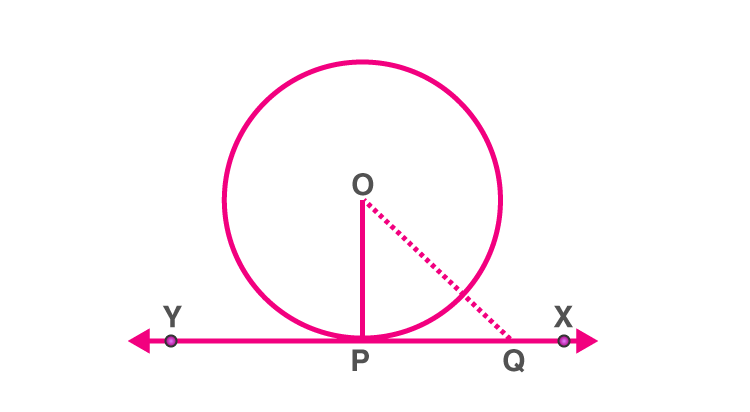
Tangent and radius
Here, O is the centre and OP⊥XY.
For More Information On Perpendicular Tangent Theorem, Watch The Below Video.

The number of tangents drawn from a given point
i) If the point is in an interior region of the circle, any line through that point will be a secant. So, no tangent can be drawn to a circle which passes through a point that lies inside it.
No tangent can be drawn to a circle from a point inside it
ii) When a point of tangency lies on the circle, there is exactly one tangent to a circle that passes through it.
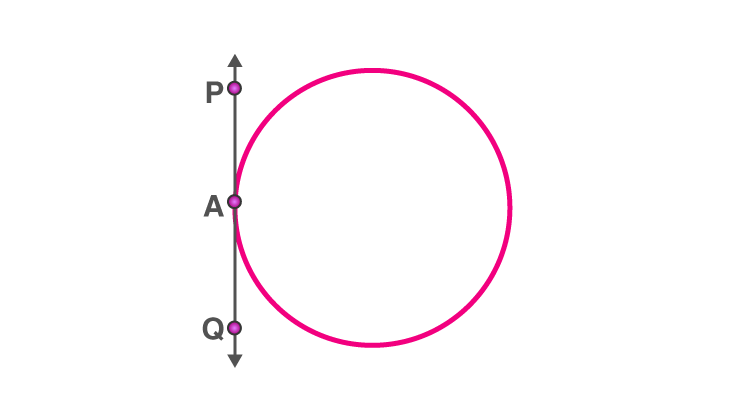
A tangent passing through a point lying on the circle
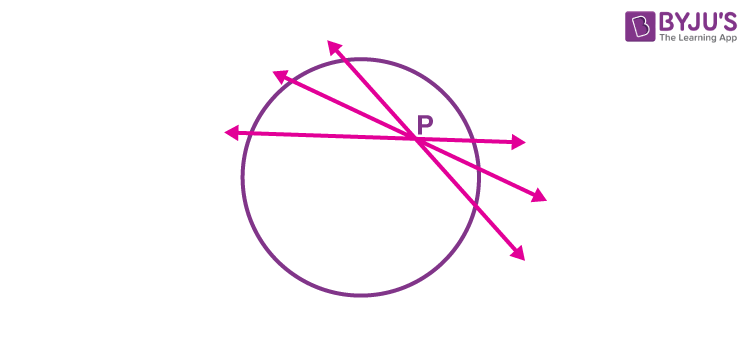
iii) When the point lies outside of the circle, there are accurately two tangents to a circle through it
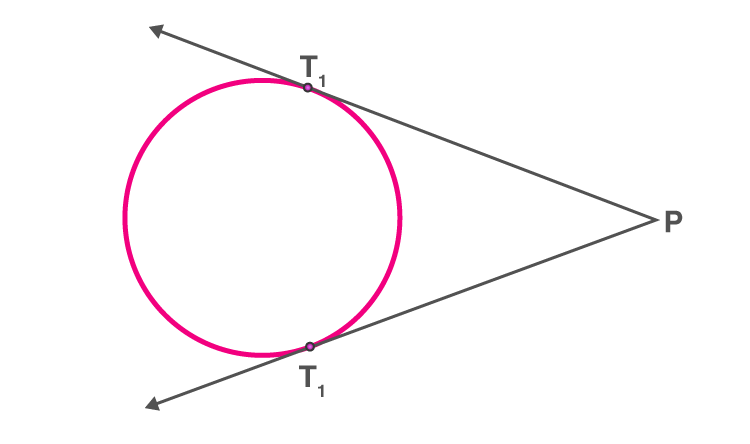
Tangents to a circle from an external point
Length of a tangent
The length of the tangent from the point (Say P) to the circle is defined as the segment of the tangent from the external point P to the point of tangency I with the circle. In this case, PI is the tangent length.
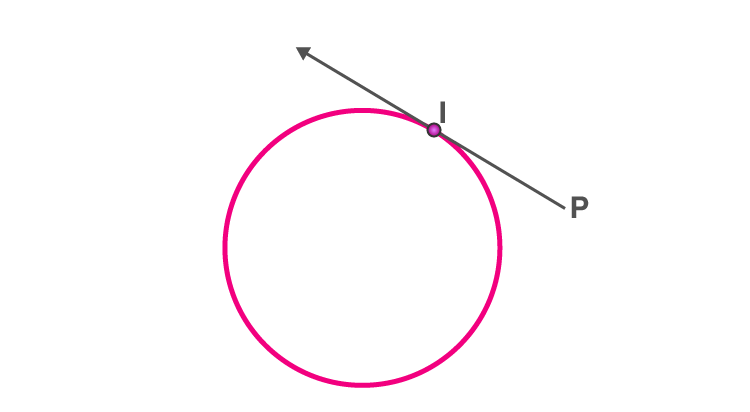
Lengths of tangents drawn from an external point
Theorem: Two tangents are of equal length when the tangent is drawn from an external point to a circle.
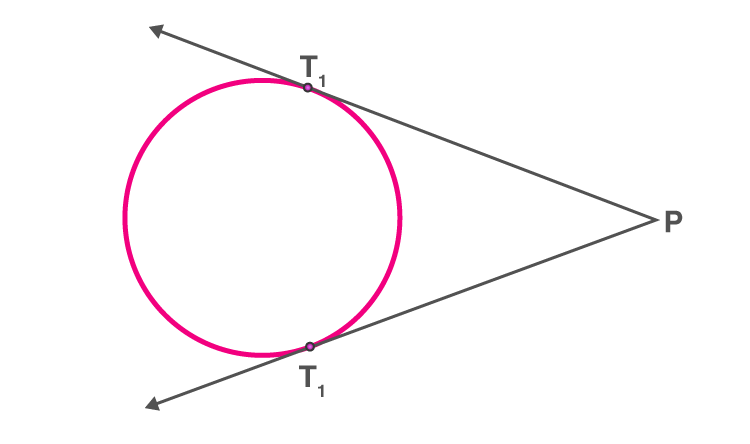
Tangents to a circle from an external point
PT1=PT2
Thus, the two important theorems in Class 10 Maths Chapter 10 Circles are:
Theorem 10.1: The tangent at any point of a circle is perpendicular to the radius through the point of contact.
Theorem 10.2: The lengths of tangents drawn from an external point to a circle are equal.
Interesting facts about Circles and its properties are listed below:
- In two concentric circles, the chord of the larger circle, which touches the smaller circle, is bisected at the point of contact.
- The tangents drawn at the ends of a diameter of a circle are parallel.
- The perpendicular at the point of contact to the tangent to a circle passes through the centre.
- The angle between the two tangents drawn from an external point to a circle is supplementary to the angle subtended by the line segment joining the points of contact at the centre.
- The parallelogram circumscribing a circle is a rhombus.
- The opposite sides of a quadrilateral circumscribing a circle subtend supplementary angles at the centre of the circle.
To know more about Tangent Circle, visit here.



Sslc maths important question and answered
https://byjus.com/maths/important-questions-class-10-maths/
Thank you for ur notes. really helpfull
it was nice, like really nice
Thank you so much for notes. It was very useful to me. ??
Thank you so much BYJUS for providing such important and helpful notes !!