In Constructions class 9 chapter 11, explains how different shapes are drawn using the compass and ruler. In this chapter, you can learn how to construct the bisector of the given angle, construction of a perpendicular bisector of the given line segment are explained with construction steps and clear diagram. Also, solve all the important questions for class 9 Maths Chapter 11 construction problems given in the textbook to score good marks.
Constructions Class 9 Concepts
The important topics and subtopics covered under constructions class 9 are:
- Introduction
- Basic constructions
- Some constructions of triangles
- Summary
Construction Class 9 Notes
For constructing the angle bisector of angle ABC, take B as a centre construct an arc (any radius) intersecting the rays BA and BC at D and E. Now take E and D as centres and radius more than ½ DE, construct arcs such that they intersect with each other at F.
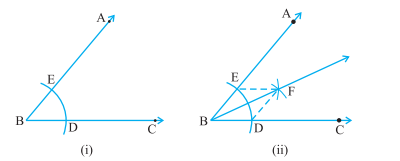
Join BF. This line is the required angle bisector of given angle ABC.
Proof:
Join EF and DF. Now, from triangle BEF and triangle BDF, BD = BE (Radius of the same arc), DF = EF (Arcs of equal radii) and BF is Common. Therefore, triangle BEF is congruent to triangle BDF by SSS congruence rule. Hence by CPCT, angle EBF is equal to angle DBF.
Constructions Class 9 Example
Go through the below example:
Example:
Given that AB is a line segment. Construct the perpendicular bisector for the given line segment.
Construction Steps:
- Take A and B as centres and radius should be more than 1/2 AB. Now, draw arcs on both sides of the line segment AB (the arc should intersect each other)
- Assume that these arcs intersect each other at the points P and Q, and join the points PQ
- Now, PQ intersect AB at the point M. Therefore, the line PMQ is the required perpendicular bisector of AB
Now, let us see how this method gives us the perpendicular bisector of AB.
To prove this, join A and B to both P and Q to form AP, AQ, BP and BQ.
From the triangles PAQ and PBQ
AP = BP and AQ = BQ (Arcs of equal radii)
PQ = PQ (Common)
Therefore, by using SSS Rule, ∆ PAQ ≅ ∆ PBQ
Thus, ∠ APM = ∠ BPM (CPCT)
Now in the triangles PMA and PMB,
AP = BP
PM = PM (Common)
∠ APM = ∠ BPM
Thus by using SAS rule, we get ∆ PMA ≅ ∆ PMB
Hence, by using CPCT, AM = BM and ∠ PMA = ∠ PMB
From the linear pair axiom ∠ PMA + ∠ PMB = 180°
∠ PMA = ∠ PMB = 90°
Therefore, the line PM, that is, PMQ is the perpendicular bisector of the line segment AB.
Practice Problems
Solve the practical problems given below for constructions class 9:
- Construct an angle of 45° at the initial point of a given ray. And also, justify the construction.
- Construct a triangle ABC in which ∠B = 30°, ∠C = 90° and AB + BC+ CA = 11 cm.
- Construct a triangle XYZ in which YZ = 8cm, ∠Y = 45° and XY – XZ= 3.5 cm
- Construct the angle 105°, and verify that using the protractor.
For more information on class 9 concepts and notes, register with BYJU’S – The Learning App and also refer NCERT, RD Sharma, RS Agarwal solutions to solve important questions.
| Related Links | |
| Algebraic Identities Class 9 | Circles Class 9 |
| Congruence of Triangles Class 9 | Coordinate Geometry Class 9 |


Comments