Cell Or Electrochemical Cell
A cell or an electrochemical cell is a device that is capable of obtaining electrical energy from chemical reactions or vice versa. You have definitely seen a cell, the small AAA or AA batteries we use in our remotes.
An electric battery is a device made up of two or more cells that make use of the chemical energy stored in the chemicals and converts it into electrical energy. A battery is used to provide a continuous steady current source by way of providing constant EMF or Electromotive force to an electrical circuit or a machine.
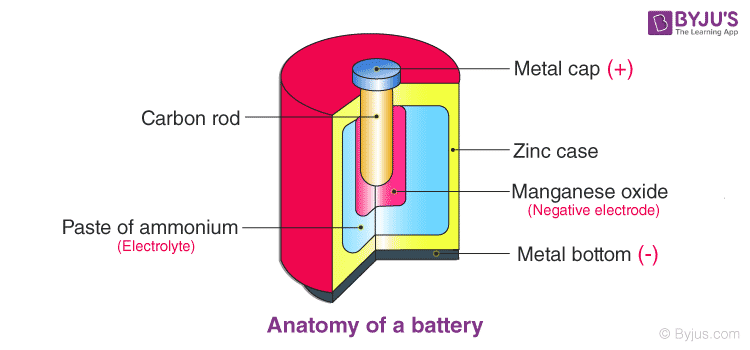
Each cell comprises two half-cells connected in series by a conductive electrolyte containing anions and cations. One half-cell is made up of the electrolyte and the negative electrode, the Anode. The negatively charged ions also known as Anions, migrate to the Anode. The other half-cell includes electrolyte and the positive electrode, the Cathode, to which cations (positively charged ions) migrate.
Redox reactions, reduction and oxidation occur simultaneously and this powers the battery. Cations are reduced (it gains electrons) at the cathode during charging, while anions are oxidized (it loses electrons) at the anode during charging. During discharge, the process is reversed. The electrodes do not touch each other but are electrically connected by the electrolyte.
What is an Electromotive Force (EMF) of a Cell?
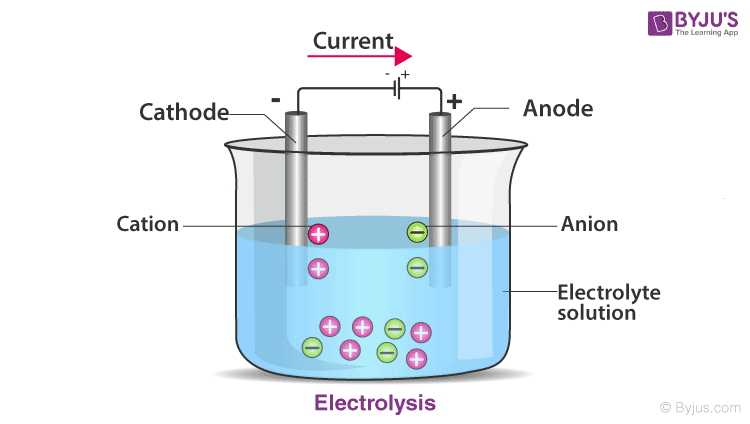
When there is no electrical equipment attached to a cell i.e. no current is flowing through the cell, the electrolyte has the same potential (emf) throughout the cell. The condition of no current flowing through a cell is also known as an open circuit. An open circuit result in a potential (emf) of the cell is equal to the difference of potentials (emf) of the electrodes. Anode has a positive potential (V+) whereas Cathode has a negative potential (-V–). This potential difference is known as the Electromotive Force (EMF) of the cell and it is equal to;
We know that when we connect an appliance to the battery, a current flows through the circuit that is proportional to the voltage. The ratio of the voltage (V) across an object to the current flowing through it because of the potential is known as the Resistance of that object. Resistance in Electricity can be linked to Friction in Mechanics. The electrical resistance of a body is the measure of the difficulty in passing an electric current through it. The SI unit of electrical resistance is ohm (Ω). The phenomenon of electrical resistance has led to the creation of electrical heaters and induction cook tops. Resistance is given by
The below video explains the relation between the EMF and voltage:
Explanation
Similar to how a body opposes the flow of electricity through it, leading to resistance, the electrolytes in batteries to have a finite value of resistance. The resistance generated inside a battery to the flow of current is referred to as the internal resistance of a battery (r). When a power source delivers current, the measured voltage output is lower than the voltage when no current is flowing through the circuit. This voltage drop is caused by the internal resistance of the battery to the flow of current through it.
The voltage drop experienced due to internal resistance can be denoted as,
This internal resistance is usually neglected in real life since the electromotive force (emf) is quite larger than the internal resistance. When external electrical equipment that uses current is attached to it, its own resistance (R) is also added to this.
Substituting this into
In this article, you learnt about a cell, battery, the electromotive force (emf) and internal resistance. If you want to learn more about EMF and cells, download BYJU’S the Learning App.
Watch an interesting video explaining current, resistance and voltage (emf).




Comments