Mirror Formula For Spherical Mirrors

An object placed in front of a mirror generates an image. If light rays from the object fall on the mirror and are then reflected and converge to form an image, the image thus formed is a real image. If the reflected light rays do not converge but have to be extrapolated backwards to form an image, the image is called a virtual image.

Looking for other study material?

In this page, we shall discuss the mirror formula, that is often taught and frequently used in various instances. As such, using ray diagrams, it is possible to determine the type of image formed, while using concave and convex mirrors, based on the distance of the object from the mirror. To obtain exact information about the size and magnification of the image, and the distance of the image from the spherical mirror, we can use the mirror formula.

The Mirror Formula (also referred to as the mirror equation) gives us the relationship between the focal length (f), the distance of the object from the mirror (u) and the distance of the image from the mirror (v). The mirror formula for a concave mirror is given below. The magnification image formed by a spherical mirror is given by the height of image divided by the height of the object. The formula is given as:

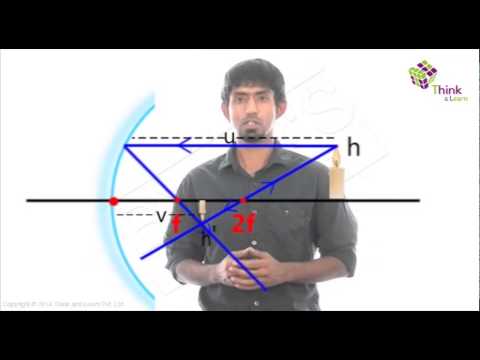
Sign Convention for Spherical Mirrors (Concave and Convex Mirrors)

• Distances are to be measured from the pole (vertex) of the mirror marked by point V in the figure.
• Distances measured along the direction of the incident ray are positive. The distance measured opposite the direction of the incident ray are negative.
• Distances measured above the principal axis are positive. Distances measured below the principal axis are negative.

The above convention applies to both concave and convex mirrors and spherical mirrors. The figure below shows a concave mirror, but the same applies for a convex mirror as well.

Following is the table explaining concepts related to mirror:

Here are some examples of spherical mirror formula problems:

1) A lens having a focal distance of 30 cm is placed in front of an object, which is located at 1 m from it. Where is an image of the object located?

Answer:

From the image position formula:

1/f = 1/o + 1/I or 1/o + 1/I = 1/f

1/(100 cm) + 1/I = 1/(30 cm)

1/I =0.023/cm

I = 42.85 cm

2) A lens forms an image at 25 cm from it, where the real object is placed at 1 m. What are the focal length of the lens and its curvature radius?

Answer:

From the image position formula, we find:

1/f = 1/o + 1/I = (o+I)/(o*I)

f = o*I/(o+I) = 25 cm * 100 cm2 /(125 cm)

f = 2500/125 cm = 20 cm

then the curvature radius is r ≈ 2 * f = 40 cm

Stay tuned with BYJU’S for more such interesting articles. Also, register to “BYJU’S – The Learning App” for loads of interactive, engaging Physics-related videos and an unlimited academic assist.